DIMENSIONS AND UNITS
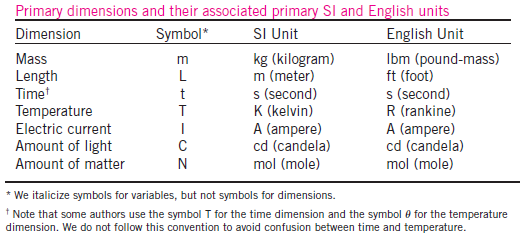
A dimension is a measure of a physical quantity (without numerical values), while a unit is a way to assign a number to that dimension. For example, length is a dimension that is measured in units such as microns ( m), feet (ft), centimeters (cm), meters (m), kilometers (km), etc. There are seven primary dimensions (also called fundamental or basic dimensions)—mass, length, time, temperature, electric current, amount of light, and amount of matter.
All nonprimary dimensions can be formed by some combination of the seven primary dimensions.
For example, force has the same dimensions as mass times acceleration (by
Newton’s second law). Thus, in terms of primary dimensions,
Dimensions of force:
where the brackets indicate “the dimensions of” and the abbreviations are taken from Table . You should be aware that some authors prefer force instead of mass as a primary dimension—we do not follow that practice.
Example : Primary Dimensions of Surface Tension An engineer is studying how some insects are able to walk on water (Fig.). A fluid property of importance in this problem is surface tension (ss), which has dimensions of force per unit length. Write the dimensions of surface tension in terms of primary dimensions.
Solution: The primary dimensions of surface tension are to be determined.
Analysis From Eq., force has dimensions of mass times acceleration, or
{mL/ t2}. Thus,
Solution: The primary dimensions of surface tension are to be determined.
Analysis From Eq., force has dimensions of mass times acceleration, or
{mL/ t2}. Thus,
Dimensions of surface tension:
Discussion The usefulness of expressing the dimensions of a variable or
constant in terms of primary dimensions will become clearer in the
discussion of the method of repeating variables in Section
constant in terms of primary dimensions will become clearer in the
discussion of the method of repeating variables in Section
0 comments